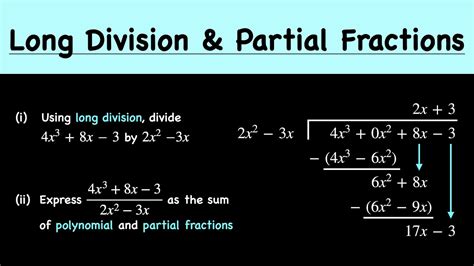
If you're a student of mathematics, chances are you've come across partial fractions long division. This is a technique used to break down rational functions into simpler fractions that are easier to work with. In this article, we'll take an in-depth look at partial fractions long division, how it works, and how you can use it to solve complex mathematical problems.
What are Rational Functions?
Before we dive into partial fractions long division, let's first define what rational functions are. A rational function is a function that can be expressed as the quotient of two polynomial functions. In other words, a rational function is a fraction where the numerator and denominator are both polynomials.
For example, the function f(x) = (x^2 + 3x + 2) / (x + 1) is a rational function because both the numerator and denominator are polynomials. Rational functions can be quite complex to work with, especially when the degree of the numerator is greater than the degree of the denominator. This is where partial fractions long division comes in handy.
What is Partial Fractions Long Division?
Partial fractions long division is a technique used to break down a complex rational function into simpler fractions. The goal is to express the original function as a sum of these simpler fractions, where each fraction has a simpler denominator than the original function. This makes it easier to integrate, differentiate, or perform any other operation on the function.
Partial fractions long division is based on the fact that any rational function can be expressed as a sum of simpler fractions, known as partial fractions. These partial fractions can be either proper or improper. A proper fraction is one where the degree of the numerator is less than the degree of the denominator, while an improper fraction is one where the degree of the numerator is equal to or greater than the degree of the denominator.

How to Perform Partial Fractions Long Division
Performing partial fractions long division involves several steps. Let's look at these steps in detail:
Step 1: Factorize the Denominator
The first step is to factorize the denominator of the rational function. This is important because it helps us to identify the simpler fractions that we need to break down the original function into. For example, let's say we have the rational function f(x) = (3x^2 + 7x + 2) / (x^2 + 3x + 2). To factorize the denominator, we need to find two numbers that multiply to give us 2 and add up to give us 3. These numbers are 1 and 2. Therefore, we can factorize the denominator as (x + 1)(x + 2).
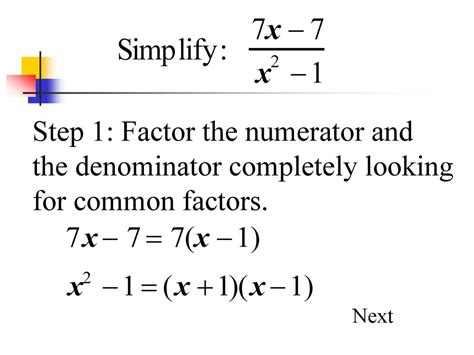
Step 2: Breakdown the Fractions
Once we've factorized the denominator, we can now break down the original fraction into simpler fractions. To do this, we need to determine the form of the partial fractions that we need. There are three forms of partial fractions:
- Proper fractions with distinct linear factors in the denominator
- Improper fractions with repeated linear factors in the denominator
- Improper fractions with quadratic factors in the denominator
Let's look at each of these forms in detail.
Proper Fractions with Distinct Linear Factors in the Denominator
If the factors in the denominator of the rational function are distinct linear factors, then the partial fractions take the form:
A / (x - a) + B / (x - b) + ... + N / (x - n)
where A, B, ..., N are constants to be determined and a, b, ..., n are the distinct linear factors in the denominator.
For example, let's look at the rational function f(x) = (3x^2 + 7x + 2) / (x + 1)(x + 2). Since the factors in the denominator are distinct linear factors, we can express the function as:
f(x) = A / (x + 1) + B / (x + 2)
where A and B are constants to be determined. To find these constants, we can use the following steps:
1. Multiply both sides of the equation by the denominator:
f(x)(x + 1)(x + 2) = A(x + 2) + B(x + 1)
2. Substitute values of x that will eliminate all but one of the unknown constants:
Let x = -1, then:
f(-1)(-1 + 1)(-1 + 2) = A(-1 + 2) + B(-1 + 1)
f(-1) = A
Let x = -2, then:
f(-2)(-2 + 1)(-2 + 2) = A(-2 + 2) + B(-2 + 1)
f(-2) = B
Now that we have determined the values of A and B, we can substitute them back into the original equation to get:
f(x) = 1 / (x + 1) - 2 / (x + 2)
This is the partial fraction decomposition of the rational function f(x).
Improper Fractions with Repeated Linear Factors in the Denominator
If the factors in the denominator of the rational function are repeated linear factors, then the partial fractions take the form:
A / (x - a) + B / (x - a)^2 + ... + N / (x - a)^n
where A, B, ..., N are constants to be determined and a is the repeated linear factor in the denominator.
For example, let's look at the rational function f(x) = (x^2 + 3x + 2) / (x + 1)^2. Since the denominator has a repeated linear factor, we can express the function as:
f(x) = A / (x + 1) + B / (x + 1)^2
where A and B are constants to be determined. To find these constants, we can use the following steps:
1. Multiply both sides of the equation by the denominator:
f(x)(x + 1)^2 = A(x + 1) + B
2. Differentiate both sides of the equation with respect to x:
f'(x)(x + 1)^2 + 2f(x)(x + 1) = A
3. Substitute x = -1 to determine the value of A:
f'(-1) = A
4. Substitute the value of A into the original equation to determine the value of B:
B = f(-1) - A
Now that we have determined the values of A and B, we can substitute them back into the original equation to get:
f(x) = -1 / (x + 1) + 4 / (x + 1)^2
This is the partial fraction decomposition of the rational function f(x).
Improper Fractions with Quadratic Factors in the Denominator
If the factors in the denominator of the rational function are quadratic factors, then the partial fractions take the form:
Ax + B / (ax^2 + bx + c) + Dx + E / (fx^2 + gx + h) + ... + Nx + P / (rx^2 + sx + t)
where A, B, ..., N and a, b, c, ..., r, s, t are constants to be determined.
For example, let's look at the rational function f(x) = (3x + 1) / (x^2 + 2x + 1). Since the denominator is a quadratic factor, we can express the function as:
f(x) = (Ax + B) / (x + 1)^2
where A and B are constants to be determined. To find these constants, we can use the following steps:
1. Multiply both sides of the equation by the denominator:
f(x)(x + 1)^2 = Ax + B
2. Substitute x = -1 to determine the value of B:
f(-1) = -A + B
3. Differentiate both sides of the equation with respect to x:
f'(x)(